How to Draw an Impossible Cube

Parallelogram to impossible cube

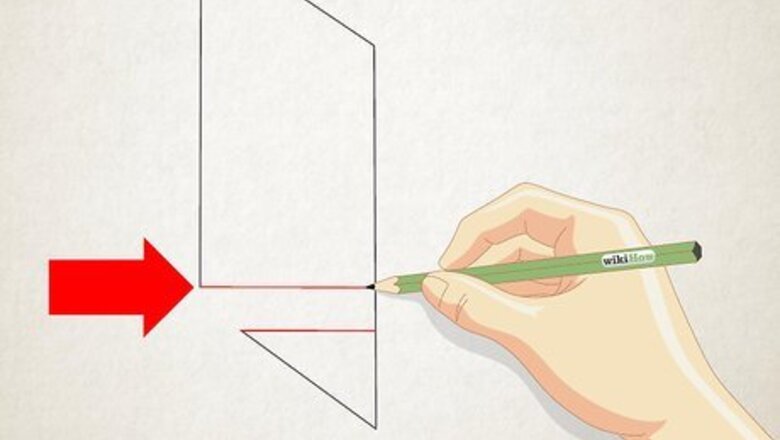
Draw a skinny, vertical parallelogram with the lower left corner open; from there, draw two lines going horizontally, shown in red.

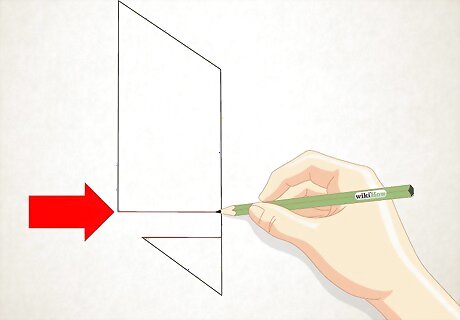
Add two connecting lines on the right side of the parallelogram. They should form an "L".

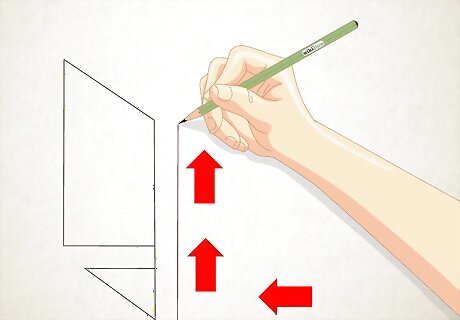
Add another two lines which continue from the corner of the parallelogram but pass beneath its right edge. The two lines then separate vertically, one going up and another going down to meet with the end of the "L".

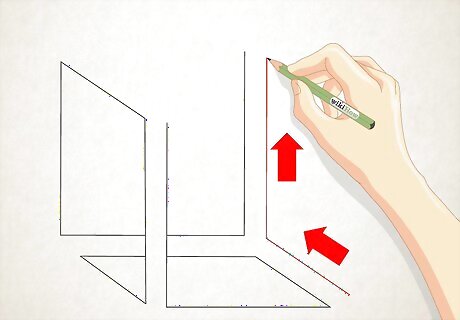
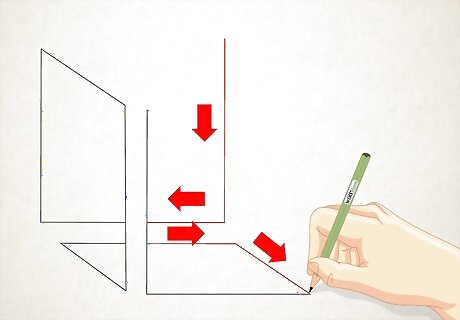
Draw a wide "L" that follows where the two previously drawn lines part ways.

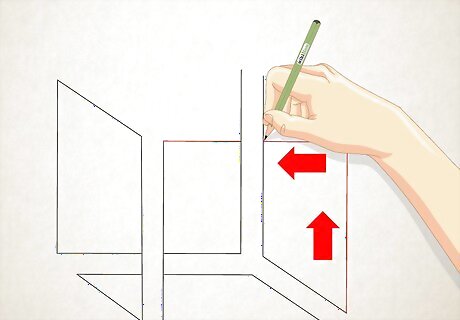
Connect the bottom end of the wide "L" with the upper right-hand corner of the parallelogram by drawing a line that goes up and then left (forming a right angle) and passes underneath any lines it comes across.

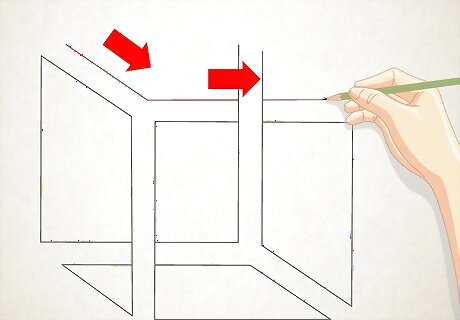
Draw a line that begins by following the top of the parallelogram and then follows the horizontal part of the line drawn in the previous step, also passing underneath any lines it comes across.

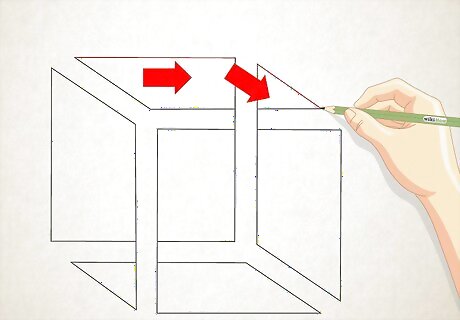
Complete a parallelogram at the top of the cube, this one with its upper right-hand corner open and connected to double lines drawn earlier.

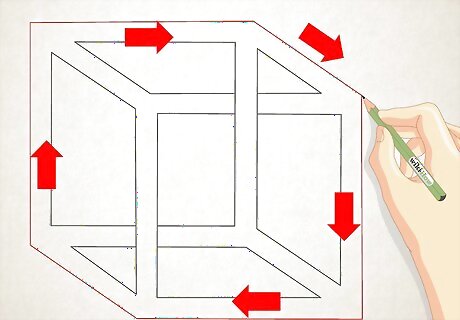
Add a border around the whole thing.

Erasing square to impossible cube

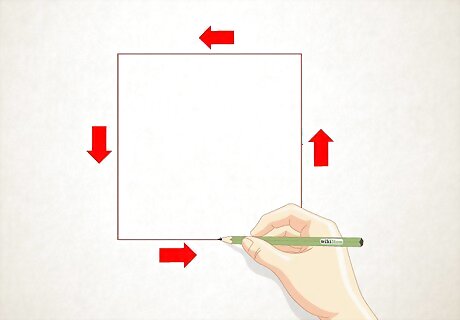
Draw a square.

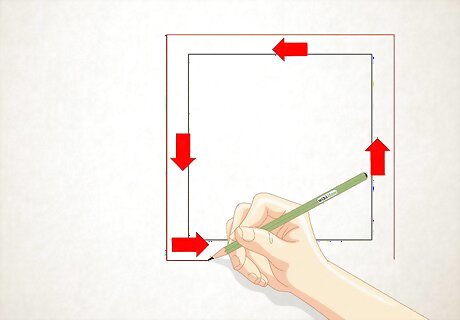
Create a slightly larger square around the first.

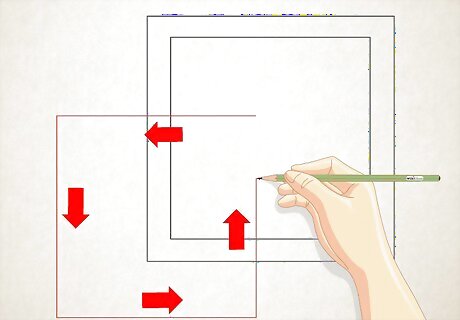
Draw another square, lower left corner in the center of the first square.

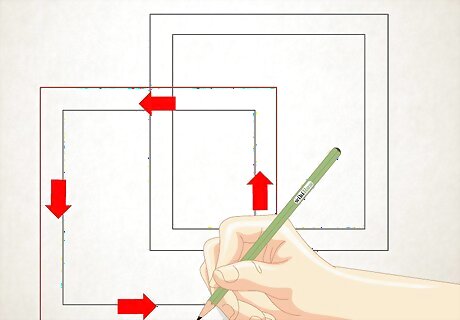
Create a square around the new square as well.

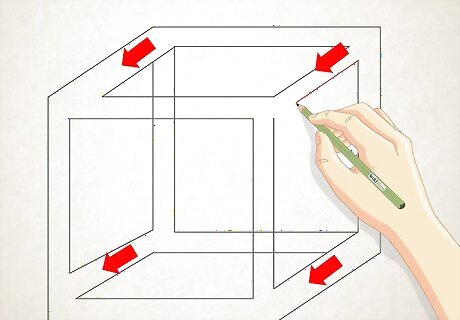
Erase each corner of both squares. Connect each corner with the same corner on the other square.

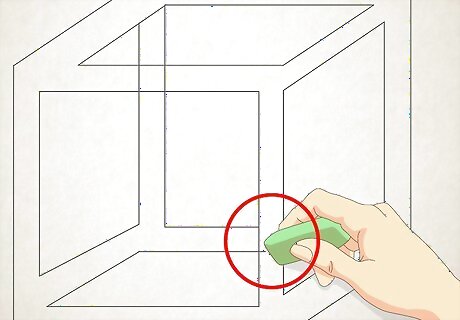
At the parts where the left of square 2 and the top of square 1 overlap each other, erase a bit to make the line horizontal, erase the vertical parts.

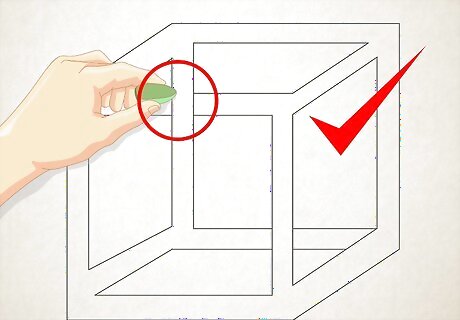
At the parts where the right of square 1 and the bottom of square 3 overlap, erase the horizontal parts and keep the vertical parts.